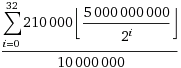Controlled supply

A fixed money supply, or a supply altered only in accord with objective and calculable criteria, is a necessary condition to a meaningful just price of money.

—Fr. Bernard W. Dempsey, S.J. (1903-1960)

In a centralized economy, currency is issued by a central bank at a rate that is supposed to match the growth of the amount of goods that are exchanged so that these goods can be traded with stable prices. The monetary base is controlled by a central bank. In the United States, the Fed increases the monetary base by issuing currency, increasing the amount banks have on reserve, and more recently, printing money electronically in a process called Quantitative Easing.

In a fully decentralized monetary system, there is no central authority that regulates the monetary base. Instead, currency is created by the nodes of a peer-to-peer network. The Bitcoin generation algorithm defines, in advance, how currency will be created and at what rate. Any currency that is generated by a malicious user that does not follow the rules will be rejected by the network and thus is worthless.

Currency with Finite Supply

Block reward halving

Controlled supply

Bitcoins are created each time a user discovers a new block. The rate of block creation is approximately constant over time: 6 per hour. The number of bitcoins generated per block is set to decrease geometrically, with a 50% reduction every four years. The result is that the number of bitcoins in existence will never exceed 21 million. Speculated justifications for the unintuitive value "21 million" are: it matches a 4-year reward halving schedule; or the ultimate total number of Satoshis that will be mined is close to the maximum capacity of a 64-bit floating point number.

This decreasing-supply algorithm was chosen because it approximates the rate at which commodities like gold are mined. Users who use their computers to perform calculations to try and discover a block are thus called Miners.

Projected Bitcoins Short Term

This chart shows the number of bitcoins that will exist in the near future. The Year is a forecast and may be slightly off.

Date reached	Block	Reward Era	BTC/block	Year (estimate)	Start BTC	BTC Added	End BTC	BTC Increase	End BTC % of Limit
2009-01-03	0	1	50.00	2009	0	2625000	2625000	infinite	12.500%
2010-04-22	52500	1	50.00	2010	2625000	2625000	5250000	100.00%	25.000%
2011-01-28	105000	1	50.00	2011	5250000	2625000	7875000	50.00%	37.500%
2011-12-14	157500	1	50.00	2012	7875000	2625000	10500000	33.33%	50.000%
2012-11-28	210000	2	25.00	2013	10500000	1312500	11812500	12.50%	56.250%
2013-10-09	262500	2	25.00	2014	11812500	1312500	13125000	11.11%	62.500%
2014-08-11	315000	2	25.00	2015	13125000	1312500	14437500	10.00%	68.750%
	367500	2	25.00	2016	14437500	1312500	15750000	9.09%	75.000%
	420000	3	12.50	2017	15750000	656250	16406250	4.17%	78.125%
	472500	3	12.50	2018	16406250	656250	17062500	4.00%	81.250%
	525000	3	12.50	2019	17062500	656250	17718750	3.85%	84.375%
	577500	3	12.50	2020	17718750	656250	18375000	3.70%	87.500%
	630000	4	6.25	2021	18375000	328125	18703125	1.79%	89.063%
	682500	4	6.25	2022	18703125	328125	19031250	1.75%	90.625%
	735000	4	6.25	2023	19031250	328125	19359375	1.72%	92.188%
	787500	4	6.25	2024	19359375	328125	19687500	1.69%	93.750%

Projected Bitcoins Long Term

Supply timeline estimation

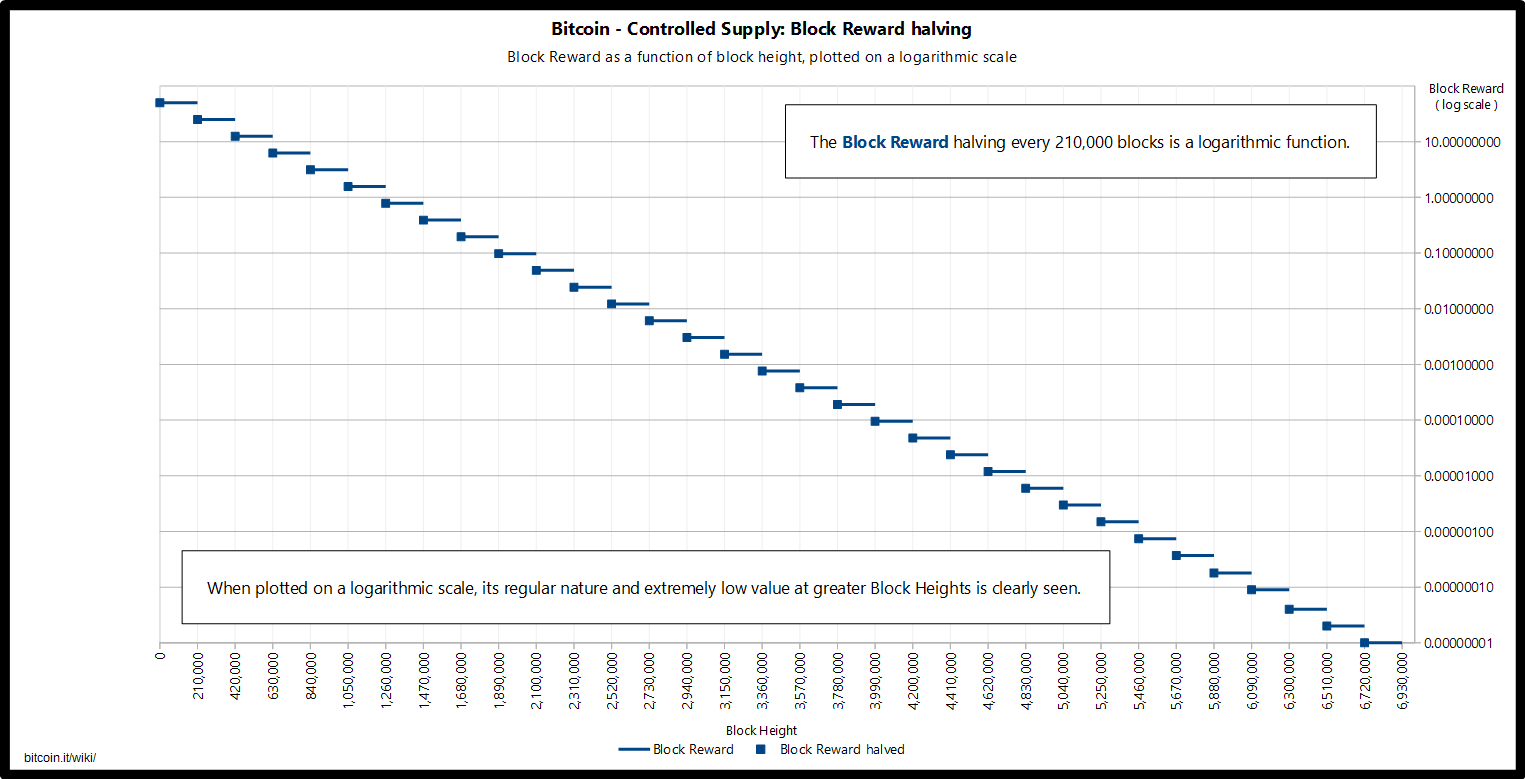
Because the number of bitcoins created each time a user discovers a new block - the block reward - is halved based on a fixed interval of blocks, and the time it takes on average to discover a block can vary based on mining power and the network difficulty, the exact time when the block reward is halved can vary as well. Consequently, the time the last Bitcoin will be created will also vary, and is subject to speculation based on assumptions.

If the mining power had remained constant since the first Bitcoin was mined, the last Bitcoin would have been mined on October 8th, 2140. Due to the mining power having increased overall over time, as of block NNNNNN, assuming mining power remains constant at that point, the last Bitcoin would be mined on May 19th, 2140.

As it is very difficulty to predict how mining power will evolve going into the future - i.e. whether technological progress will continue to make hardware faster or whether mining will hit a a technological wall, or whether or not faster methods of calculation will be discovered - putting an exact date or even year on this event is difficult as well.

The total number of bitcoins, as mentioned earlier, will tend toward 21 million. Due to a technical limitation in the data structure of the blockchain - specifically of the transaction output, this exact value is 20,999,999.9769 bitcoin. Should this technical limitation is adjusted, the total number will still only be a maximum of 21 million.

Block	Reward Era	BTC/block	Year	Start BTC	BTC Added	End BTC	BTC Increase	End BTC % of Limit
0	1	50.00000000	2009.007	0.00000000	10500000.00000000	10500000.00000000	infinite	50.00000006%
210000	2	25.00000000	2013.000	10500000.00000000	5250000.00000000	15750000.00000000	50.00000000%	75.00000008%
420000	3	12.50000000	2016.993	15750000.00000000	2625000.00000000	18375000.00000000	16.66666667%	87.50000010%
630000	4	6.25000000	2020.986	18375000.00000000	1312500.00000000	19687500.00000000	7.14285714%	93.75000010%
840000	5	3.12500000	2024.978	19687500.00000000	656250.00000000	20343750.00000000	3.33333333%	96.87500011%
1050000	6	1.56250000	2028.971	20343750.00000000	328125.00000000	20671875.00000000	1.61290323%	98.43750011%
1260000	7	0.78125000	2032.964	20671875.00000000	164062.50000000	20835937.50000000	0.79365079%	99.21875011%
1470000	8	0.39062500	2036.956	20835937.50000000	82031.25000000	20917968.75000000	0.39370079%	99.60937511%
1680000	9	0.19531250	2040.949	20917968.75000000	41015.62500000	20958984.37500000	0.19607843%	99.80468761%
1890000	10	0.09765625	2044.942	20958984.37500000	20507.81250000	20979492.18750000	0.09784736%	99.90234386%
2100000	11	0.04882812	2048.934	20979492.18750000	10253.90520000	20989746.09270000	0.04887585%	99.95117198%
2310000	12	0.02441406	2052.927	20989746.09270000	5126.95260000	20994873.04530000	0.02442599%	99.97558604%
2520000	13	0.01220703	2056.920	20994873.04530000	2563.47630000	20997436.52160000	0.01221001%	99.98779307%
2730000	14	0.00610351	2060.913	20997436.52160000	1281.73710000	20998718.25870000	0.00610426%	99.99389658%
2940000	15	0.00305175	2064.905	20998718.25870000	640.86750000	20999359.12620000	0.00305194%	99.99694833%
3150000	16	0.00152587	2068.898	20999359.12620000	320.43270000	20999679.55890000	0.00152592%	99.99847420%
3360000	17	0.00076293	2072.891	20999679.55890000	160.21530000	20999839.77420000	0.00076294%	99.99923713%
3570000	18	0.00038146	2076.883	20999839.77420000	80.10660000	20999919.88080000	0.00038146%	99.99961859%
3780000	19	0.00019073	2080.876	20999919.88080000	40.05330000	20999959.93410000	0.00019073%	99.99980932%
3990000	20	0.00009536	2084.869	20999959.93410000	20.02560000	20999979.95970000	0.00009536%	99.99990468%
4200000	21	0.00004768	2088.861	20999979.95970000	10.01280000	20999989.97250000	0.00004768%	99.99995236%
4410000	22	0.00002384	2092.854	20999989.97250000	5.00640000	20999994.97890000	0.00002384%	99.99997620%
4620000	23	0.00001192	2096.847	20999994.97890000	2.50320000	20999997.48210000	0.00001192%	99.99998812%
4830000	24	0.00000596	2100.840	20999997.48210000	1.25160000	20999998.73370000	0.00000596%	99.99999408%
5040000	25	0.00000298	2104.832	20999998.73370000	0.62580000	20999999.35950000	0.00000298%	99.99999706%
5250000	26	0.00000149	2108.825	20999999.35950000	0.31290000	20999999.67240000	0.00000149%	99.99999855%
5460000	27	0.00000074	2112.818	20999999.67240000	0.15540000	20999999.82780000	0.00000074%	99.99999929%
5670000	28	0.00000037	2116.810	20999999.82780000	0.07770000	20999999.90550000	0.00000037%	99.99999966%
5880000	29	0.00000018	2120.803	20999999.90550000	0.03780000	20999999.94330000	0.00000018%	99.99999984%
6090000	30	0.00000009	2124.796	20999999.94330000	0.01890000	20999999.96220000	0.00000009%	99.99999993%
6300000	31	0.00000004	2128.788	20999999.96220000	0.00840000	20999999.97060000	0.00000004%	99.99999997%
6510000	32	0.00000002	2132.781	20999999.97060000	0.00420000	20999999.97480000	0.00000002%	99.99999999%
6720000	33	0.00000001	2136.774	20999999.97480000	0.00210000	20999999.97690000	0.00000001%	100.00000000%
6930000	34	0.00000000	2140.767	20999999.97690000	0.00000000	20999999.97690000	0.00000000%	100.00000000%

Note: The number of bitcoins are presented in a floating point format. However, these values are based on the number of satoshi per block originally in integer format to prevent compounding error.

Spendable Supply

The theoretical total number of bitcoins, 21 million, should not be confused with the total spendable supply. The total spendable supply is always lower than the theoretical total supply, and is subject to accidental loss, willful destruction, and technical peculiarities.

Loss of bitcoin

Bitcoins may be lost if the conditions required to spend them are no longer known. For example, if you made a transaction to an address that requires a private key in order to spend those bitcoins further, had written that private key down on a piece of paper, but that piece of paper was lost. In this case, that bitcoin may also be considered lost, as the odds of randomly finding a matching private key are such that it is generally considered impossible.

Willful destruction of bitcoin

Bitcoins may also be willfully 'destroyed' - for example by attaching conditions that make it impossible to spend them.

A common method is to send bitcoin to an address that was constructed and only made to pass validity checks, but for which no private key is actually known. An example of such an address is "1BitcoinEaterAddressDontSendf59kuE", where the last "f59kuE" is text to make the preceding constructed text pass validation. Finding a matching private key is, again, generally considered impossible. For an example of how difficult this would be, see Vanitygen.

Another common method is to send bitcoin in a transaction where the conditions for spending are not just unfathomably unlikely, but literally impossible to meet. For example, a transaction that is made provably unspendable using OP_RETURN, or uses script operations that requires the user to prove that 1+1 equals 3.

A lesser known method is to send bitcoin to an address based on private key that is outside the range of valid ECDSA private keys. For example, the address 16QaFeudRUt8NYy2yzjm3BMvG4xBbAsBFM has a known matching private key of value 0 (zero), which is outside the valid range.

Technical peculiarities preventing spending of bitcoin

There are also technical peculiarities that prevent the spending of some bitcoin.

The first 50 bitcoin, included in the genesis block, cannot be spent as its transaction is not in the global database.

Money Supply

While the number of bitcoins in existence will never exceed 21 million, the money supply of bitcoins can exceed 21 million due to Fractional-reserve Banking.

Deflation

Because the monetary base of bitcoins cannot be expanded, the currency would be subject to severe deflation if it becomes widely used. Keynesian economists argue that deflation is bad for an economy because it incentivises individuals and businesses to save money rather than invest in businesses and create jobs. The Austrian school of thought counters this criticism, claiming that as deflation occurs in all stages of production, entrepreneurs who invest benefit from it. As a result, profit ratios tend to stay the same and only their magnitudes change. In other words, in a deflationary environment, goods and services decrease in price, but at the same time the cost for the production of these goods and services tend to decrease proportionally, effectively not affecting profits. Price deflation encourages an increase in hoarding — hence savings — which in turn tends to lower interest rates and increase the incentive for entrepreneurs to invest in projects of longer term.

See also

• Milton Friedman interview, where he proposed to replace the central bank with a computer, and to fix the money supply growth at 4% annually
• Deflationary spiral
• Chart of total bitcoins in circulation